If there is one prayer that you should pray/sing every day and every hour, it is the LORD's prayer (Our FATHER in Heaven prayer)
It is the most powerful prayer. A pure heart, a clean mind, and a clear conscience is necessary for it. - Samuel Dominic Chukwuemeka

For in GOD we live, and move, and have our being. - Acts 17:28

The Joy of a Teacher is the Success of his Students. - Samuel Chukwuemeka

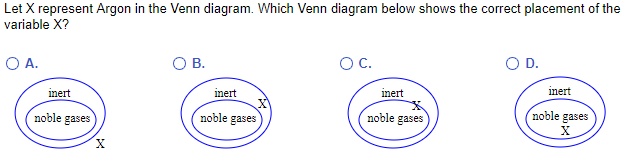
Solved Examples on Symbolic Arguments and Syllogism

Samuel Dominic Chukwuemeka (SamDom For Peace) Determine whether these arguments are valid or invalid. Show all work.

For symbolic arguments, use at least two methods: by Definition, by Formula and/or by the Valid Forms of Arguments and Invalid Forms of Arguments for each argument as applicable.

For syllogistic arguments, use Euler diagrams or Venn diagrams as applicable.

(1.)

$ p \leftrightarrow q \\[2ex] p \underline{\lor} q \\ \rule{1.2in}{0.3pt} \\ \therefore \neg q $


$ Premise\: 1: p \leftrightarrow q \\[2ex] Premise\: 2: p \underline{\lor} q \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore \neg q \\[3ex] $ Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $p \leftrightarrow q$ $p \underline{\lor} q$ $\neg q$
$T$ $T$ $T$ $F$ $F$
$T$ $F$ $F$ $T$ $T$
$F$ $T$ $F$ $T$ $F$
$F$ $F$ $T$ $F$ $T$
Premise 1 Premise 2 Conclusion
There is no case where both premises are true.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \leftrightarrow q) \land (p \underline{\lor} q)] \rightarrow \neg q$
$p$ $q$ $p \leftrightarrow q$ $p \underline{\lor} q)$ $(p \rightarrow q) \land (p \underline{\lor} q)$ $\neg q$ $[(p \rightarrow q) \land (p \underline{\lor} q)] \rightarrow \neg q$
$T$ $T$ $T$ $F$ $F$ $F$ $T$
$T$ $F$ $F$ $T$ $F$ $T$ $T$
$F$ $T$ $F$ $T$ $F$ $F$ $T$
$F$ $F$ $T$ $F$ $F$ $T$ $T$
The formula is a tautology.
Argument is valid by Formula

(2.)

$ p \lor q \\[2ex] p \\ \rule{1.2in}{0.3pt} \\ \therefore \neg q $


$ Premise\: 1: p \lor q \\[2ex] Premise\: 2: p \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore \neg q \\[3ex] $ First Method: Valid and Invalid Forms of Arguments
Argument is invalid by Misuse of Disjunctive Reasoning

Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $p \lor q$ $\neg q$
$T\checkmark$ $T$ $T\checkmark$ $F\times$
$T\checkmark$ $F$ $T\checkmark$ $T\checkmark$
$F$ $T$ $T$ $F$
$F$ $F$ $F$ $T$
Premise 2 Premise 1 Conclusion
In the $1st$ case, the conclusion is false when both premises are true.
Argument is invalid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \lor q) \land p] \rightarrow \neg q$
$p$ $q$ $p \lor q$ $(p \lor q) \land p$ $\neg q$ $[(p \lor q) \land p] \rightarrow \neg q$
$T$ $T$ $T$ $T$ $F$ $F$
$T$ $F$ $T$ $T$ $T$ $T$
$F$ $T$ $T$ $F$ $F$ $T$
$F$ $F$ $F$ $F$ $T$ $T$
The formula is not a tautology.
Argument is invalid by Formula

(3.)

$ p \rightarrow \neg q \\[2ex] q \\ \rule{1.2in}{0.3pt} \\ \therefore \neg p $


$ Premise\: 1: p \rightarrow \neg q \\[2ex] Premise\: 2: q \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore \neg p \\[3ex] $ First Method: Valid and Invalid Forms of Arguments
Argument is valid by Modus Tollens

Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $\neg q$ $p \rightarrow \neg q$ $\neg p$
$T$ $T$ $F$ $F$ $F$
$T$ $F$ $T$ $T$ $F$
$F$ $T\checkmark$ $F$ $T\checkmark$ $T\checkmark$
$F$ $F$ $T$ $T$ $T$
Premise 2 Premise 1 Conclusion

In all the cases when both premises are true (3rd case), the conclusion is also true.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \rightarrow \neg q) \land q] \rightarrow \neg p$
$p$ $q$ $\neg q$ $p \rightarrow \neg q$ $(p \rightarrow \neg q) \land q$ $\neg p$ $[(p \rightarrow \neg q) \land q] \rightarrow \neg p$
$T$ $T$ $F$ $F$ $F$ $F$ $T$
$T$ $F$ $T$ $T$ $F$ $F$ $T$
$F$ $T$ $F$ $T$ $T$ $T$ $T$
$F$ $F$ $T$ $T$ $F$ $T$ $T$

The formula is a tautology.
Argument is valid by Formula

(4.)

$ p \lor q \\[2ex] \neg p \\ \rule{1.2in}{0.3pt} \\ \therefore q $


$ Premise\: 1: p \lor q \\[2ex] Premise\: 2: \neg p \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore q \\[3ex] $ First Method: Valid and Invalid Forms of Arguments
Argument is valid by Disjunctive Reasoning

Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $p \lor q$ $\neg p$
$T$ $T$ $T$ $F$
$T$ $F$ $T$ $F$
$F$ $T\checkmark$ $T\checkmark$ $T\checkmark$
$F$ $F$ $F$ $T$
Conclusion Premise 1 Premise 2

The conclusion is true when both premises are true.
Yes, it is only one case: Case 3...that is fine.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \lor q) \land \neg p] \rightarrow q$
$p$ $q$ $p \lor q$ $\neg p$ $(p \lor q) \land \neg p$ $[(p \lor q) \land \neg p] \rightarrow q$
$T$ $T$ $T$ $F$ $F$ $T$
$T$ $F$ $T$ $F$ $F$ $T$
$F$ $T$ $T$ $T$ $T$ $T$
$F$ $F$ $F$ $T$ $F$ $T$
The formula is a tautology.
Argument is valid by Formula

(5.)

$ (p \lor q) \rightarrow r \\[2ex] \rule{1.7in}{0.3pt} \\ \therefore (p \land q) \rightarrow r $


$ Premise\: 1: (p \lor q) \rightarrow r \\[2ex] \rule{3.1in}{0.3pt} \\ Conclusion\: \therefore (p \land q) \rightarrow r \\[3ex] $ Second Method: Definition
Let us draw a truth table of the premise and the conclusion.
$p$ $q$ $r$ $p \lor q$ $(p \lor q) \rightarrow r$ $p \land q$ $(p \land q) \rightarrow r$
$T$ $T$ $T$ $T$ $T\checkmark$ $T$ $T\checkmark$
$T$ $T$ $F$ $T$ $F$ $T$ $F$
$T$ $F$ $T$ $T$ $T\checkmark$ $F$ $T\checkmark$
$T$ $F$ $F$ $T$ $F$ $F$ $T$
$F$ $T$ $T$ $T$ $T\checkmark$ $F$ $T\checkmark$
$F$ $T$ $F$ $T$ $F$ $F$ $T$
$F$ $F$ $T$ $F$ $T\checkmark$ $F$ $T\checkmark$
$F$ $F$ $F$ $F$ $T\checkmark$ $F$ $T\checkmark$
Premise 1 Conclusion
The conclusion is true whenever the premise is true.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $premise\: 1 \rightarrow conclusion$
$[(p \lor q) \rightarrow r] \rightarrow [(p \land q) \rightarrow r]$
$p$ $q$ $r$ $p \lor q$ $(p \lor q) \rightarrow r$ $p \land q$ $(p \land q) \rightarrow r$ $[(p \lor q) \rightarrow r] \rightarrow [(p \land q) \rightarrow r]$
$T$ $T$ $T$ $T$ $T$ $T$ $T$ $T$
$T$ $T$ $F$ $T$ $F$ $T$ $F$ $T$
$T$ $F$ $T$ $T$ $T$ $F$ $T$ $T$
$T$ $F$ $F$ $T$ $F$ $F$ $T$ $T$
$F$ $T$ $T$ $T$ $T$ $F$ $T$ $T$
$F$ $T$ $F$ $T$ $F$ $F$ $T$ $T$
$F$ $F$ $T$ $F$ $T$ $F$ $T$ $T$
$F$ $F$ $F$ $F$ $T$ $F$ $T$ $T$
The formula is a tautology.
Argument is valid by Formula

(6.)

$ p \land q \\[2ex] \rule{1.2in}{0.3pt} \\ \therefore p $


$ Premise\: 1: p \land q \\[2ex] \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore p \\[3ex] $ First Method: Valid and Invalid Forms of Arguments
Argument is valid by Simplification

Second Method: Definition
Let us draw a truth table of the premise and the conclusion.
$p$ $q$ $p \land q$
$T\checkmark$ $T$ $T\checkmark$
$T$ $F$ $F$
$F$ $T$ $F$
$F$ $F$ $F$
Conclusion Premise 1
The conclusion is true whenever the premise is true.
Yes, it is only one case: Case $1$...that is fine.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $premise\: 1 \rightarrow conclusion$
$(p \land q) \rightarrow p$
$p$ $q$ $p \land q$ $(p \land q) \rightarrow p$
$T$ $T$ $T$ $T$
$T$ $F$ $F$ $T$
$F$ $T$ $F$ $T$
$F$ $F$ $F$ $T$
The formula is a tautology.
Argument is valid by Formula

(7.) If yesterday was Sunday, then today is not Saturday.
Today is Saturday.
Therefore, yesterday was not Sunday.


Let:
$p$: Yesterday was Sunday.
$q$: Today is Saturday.

$ Premise\: 1: p \rightarrow \neg q \\[2ex] Premise\: 2: q \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore \neg p \\[3ex] $ First Method: Valid and Invalid Forms of Arguments
Argument is valid by Modus Tollens

Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $\neg q$ $p \rightarrow \neg q$ $\neg p$
$T$ $T$ $F$ $F$ $F$
$T$ $F$ $T$ $T$ $F$
$F$ $T\checkmark$ $F$ $T\checkmark$ $T\checkmark$
$F$ $F$ $T$ $T$ $T$
Premise 2 Premise 1 Conclusion

In all the cases when both premises are true (3rd case), the conclusion is also true.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \rightarrow \neg q) \land q] \rightarrow \neg p$
$p$ $q$ $\neg q$ $p \rightarrow \neg q$ $(p \rightarrow \neg q) \land q$ $\neg p$ $[(p \rightarrow \neg q) \land q] \rightarrow \neg p$
$T$ $T$ $F$ $F$ $F$ $F$ $T$
$T$ $F$ $T$ $T$ $F$ $F$ $T$
$F$ $T$ $F$ $T$ $T$ $T$ $T$
$F$ $F$ $T$ $T$ $F$ $T$ $T$

The formula is a tautology.
Argument is valid by Formula

(8.) If we permit expanded fracking, then there will be more natural gas available.
There is more natural gas available.

We permitted expanded fracking.


Let:
$p$: We permit expanded fracking.
$q$: There will be more natural gas available.

$ Premise\: 1: p \rightarrow q \\[2ex] Premise\: 2: q \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore p \\[3ex] $ First Method: Valid and Invalid Forms of Arguments
Argument is invalid by Fallacy of the Converse

Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $p \rightarrow q$
$T\checkmark$ $T\checkmark$ $T\checkmark$
$T$ $F$ $F$
$F\times$ $T\checkmark$ $T\checkmark$
$F$ $F$ $T$
Conclusion Premise 2 Premise 1
In the 3rd case, the conclusion is false when both premises are true.
Argument is invalid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \rightarrow q) \land q] \rightarrow p$
$p$ $q$ $p \rightarrow q$ $(p \rightarrow q) \land q$ $[(p \rightarrow q) \land q] \rightarrow p$
$T$ $T$ $T$ $T$ $T$
$T$ $F$ $F$ $F$ $T$
$F$ $T$ $T$ $T$ $F$
$F$ $F$ $T$ $F$ $T$
The formula is not a tautology.
Argument is invalid by Formula

(9.)

$ p \leftrightarrow q \\[2ex] q \rightarrow r \\ \rule{1.2in}{0.3pt} \\ \therefore \neg r \rightarrow \neg p $


$ Premise\: 1: p \leftrightarrow q \\[2ex] Premise\: 2: q \rightarrow r \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore \neg r \rightarrow \neg p \\[3ex] $ Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $r$ $p \leftrightarrow q$ $q \rightarrow r$ $\neg r$ $\neg p$ $\neg r \rightarrow \neg p$
$T$ $T$ $T$ $T\checkmark$ $T\checkmark$ $F$ $F$ $T\checkmark$
$T$ $T$ $F$ $T$ $F$ $T$ $F$ $F$
$T$ $F$ $T$ $F$ $T$ $F$ $F$ $T$
$T$ $F$ $F$ $F$ $T$ $T$ $F$ $F$
$F$ $T$ $T$ $F$ $T$ $F$ $T$ $T$
$F$ $T$ $F$ $F$ $F$ $T$ $T$ $T$
$F$ $F$ $T$ $T\checkmark$ $T\checkmark$ $F$ $T$ $T\checkmark$
$F$ $F$ $F$ $T\checkmark$ $T\checkmark$ $T$ $T$ $T\checkmark$
Premise 1 Premise 2 Conclusion

In all the cases when both premises are true, the conclusion is also true.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \rightarrow \neg q) \land q] \rightarrow \neg p$
$p$ $q$ $r$ $p \leftrightarrow q$ $q \rightarrow r$ $\neg r$ $\neg p$ $\neg r \rightarrow \neg p$ $(p \leftrightarrow q) \land (q \rightarrow r)$ $[(p \leftrightarrow q) \land (q \rightarrow r)] \rightarrow (\neg r \rightarrow \neg p)$
$T$ $T$ $T$ $T$ $T$ $F$ $F$ $T$ $T$ $T$
$T$ $T$ $F$ $T$ $F$ $T$ $F$ $F$ $F$ $T$
$T$ $F$ $T$ $F$ $T$ $F$ $F$ $T$ $F$ $T$
$T$ $F$ $F$ $F$ $T$ $T$ $F$ $F$ $F$ $T$
$F$ $T$ $T$ $F$ $T$ $F$ $T$ $T$ $F$ $T$
$F$ $T$ $F$ $F$ $F$ $T$ $T$ $T$ $F$ $T$
$F$ $F$ $T$ $T$ $T$ $F$ $T$ $T$ $T$ $T$
$F$ $F$ $F$ $T$ $T$ $T$ $T$ $T$ $T$ $T$

The formula is a tautology.
Argument is valid by Formula

(10.) You must eat well or you will not be healthy.
I eat well.
Therefore, I am healthy.


Let:
$p$: You must eat well.
$q$: You will be healthy.

$ Premise\: 1: p \lor \neg q \\[2ex] Premise\: 2: p \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore q \\[3ex] $ First Method: Valid and Invalid Forms of Arguments
Argument is invalid by Misuse of Disjunctive Reasoning

Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $\neg q$ $p \lor \neg q$
$T\checkmark$ $T\checkmark$ $F$ $T\checkmark$
$T\checkmark$ $F\times$ $T$ $T\checkmark$
$F$ $T$ $F$ $F$
$F$ $F$ $T$ $T$
Premise 2 Conclusion Premise 1
In the 2nd case, the conclusion is false when both premises are true.
Argument is invalid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \lor \neg q) \land p] \rightarrow q$
$p$ $q$ $\neg q$ $p \lor \neg q$ $(p \lor \neg q) \land p$ $[(p \lor \neg q) \land p] \rightarrow q$
$T$ $T$ $F$ $T$ $T$ $T$
$T$ $F$ $T$ $T$ $T$ $F$
$F$ $T$ $F$ $F$ $F$ $T$
$F$ $F$ $T$ $T$ $F$ $T$
The formula is not a tautology.
Argument is invalid by Formula

(11.) Premise: If you live in Baltimore, then you live in Maryland.
Premise: Amanda does not live in Baltimore.
Conclusion: Amanda does not live in Maryland.


Let:
$p$: Amanda lives in Baltimore.
$q$: Amanda lives in Maryland.

$ Premise\: 1: p \rightarrow q \\[2ex] Premise\: 2: \neg p \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore \neg q \\[3ex] $ First Method: Valid and Invalid Forms of Arguments
Argument is invalid by Fallacy of the Inverse

Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $p \rightarrow q$ $\neg p$ $\neg q$
$T$ $T$ $T$ $F$ $F$
$T$ $F$ $F$ $F$ $T$
$F$ $T$ $T\checkmark$ $T\checkmark$ $F\times$
$F$ $F$ $T\checkmark$ $T\checkmark$ $T\checkmark$
Premise 1 Premise 2 Conclusion
In the 3rd case, the conclusion is false when both premises are true.
Argument is invalid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \rightarrow q) \land \neg p] \rightarrow \neg q$
$p$ $q$ $p \rightarrow q$ $\neg p$ $\neg q$ $(p \rightarrow q) \land \neg p$ $[(p \rightarrow q) \land \neg p] \rightarrow \neg q$
$T$ $T$ $T$ $F$ $F$ $F$ $T$
$T$ $F$ $F$ $F$ $T$ $F$ $T$
$F$ $T$ $T$ $T$ $F$ $T$ $F$
$F$ $F$ $T$ $T$ $T$ $T$ $T$
The formula is not a tautology.
Argument is invalid by Formula

(12.) We must build a hydroelectric plant or a nuclear plant.
We won't build a nuclear plant, so we must build a hydroelectric plant.


Let:
$p$: We must build a hydroelectric plant.
$q$: We must build a nuclear plant.

$ Premise\: 1: p \lor q \\[2ex] Premise\: 2: \neg q \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore p \\[3ex] $ First Method: Valid and Invalid Forms of Arguments
Argument is valid by Disjunctive Reasoning

Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $p \lor q$ $\neg q$
$T$ $T$ $T$ $F$
$T\checkmark$ $F$ $T\checkmark$ $T\checkmark$
$F$ $T$ $T$ $F$
$F$ $F$ $F$ $T$
Conclusion Premise 1 Premise 2

The conclusion is true when both premises are true.
Yes, it is only one case: Case 2...that is fine.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \lor q) \land \neg q] \rightarrow p$
$p$ $q$ $p \lor q$ $\neg q$ $(p \lor q) \land \neg q$ $[(p \lor q) \land \neg q] \rightarrow p$
$T$ $T$ $T$ $F$ $F$ $T$
$T$ $F$ $T$ $T$ $T$ $T$
$F$ $T$ $T$ $F$ $F$ $T$
$F$ $F$ $F$ $T$ $F$ $T$

The formula is a tautology.
Argument is valid by Formula

(13.)

$ p \rightarrow q \\[2ex] q \land r \\ \rule{1.2in}{0.3pt} \\ \therefore p \lor r $


$ Premise\: 1: p \rightarrow q \\[2ex] Premise\: 2: q \land r \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore p \lor r \\[3ex] $ Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $r$ $p \rightarrow q$ $q \land r$ $p \lor r$
$T$ $T$ $T$ $T\checkmark$ $T\checkmark$ $T\checkmark$
$T$ $T$ $F$ $T$ $F$ $T$
$T$ $F$ $T$ $F$ $F$ $T$
$T$ $F$ $F$ $F$ $F$ $T$
$F$ $T$ $T$ $T\checkmark$ $T\checkmark$ $T\checkmark$
$F$ $T$ $F$ $T$ $F$ $F$
$F$ $F$ $T$ $T$ $F$ $T$
$F$ $F$ $F$ $T$ $F$ $F$
Premise 1 Premise 2 Conclusion

In all the cases when both premises are true, the conclusion is also true.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \rightarrow \neg q) \land q] \rightarrow \neg p$
$p$ $q$ $r$ $p \rightarrow q$ $q \land r$ $p \lor r$ $(p \rightarrow q) \land (q \land r)$ $[(p \rightarrow q) \land (q \land r)] \rightarrow (p \lor r)$
$T$ $T$ $T$ $T$ $T$ $T$ $T$ $T$
$T$ $T$ $F$ $T$ $F$ $T$ $F$ $T$
$T$ $F$ $T$ $F$ $F$ $T$ $F$ $T$
$T$ $F$ $F$ $F$ $F$ $T$ $F$ $T$
$F$ $T$ $T$ $T$ $T$ $T$ $T$ $T$
$F$ $T$ $F$ $T$ $F$ $F$ $F$ $T$
$F$ $F$ $T$ $T$ $F$ $T$ $F$ $T$
$F$ $F$ $F$ $T$ $F$ $F$ $F$ $T$

The formula is a tautology.
Argument is valid by Formula

(14.)

$ p \rightarrow q \\[2ex] \neg p \\ \rule{1.2in}{0.3pt} \\ \therefore q $


$ Premise\: 1: p \rightarrow q \\[2ex] Premise\: 2: \neg p \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore q \\[3ex] $ Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $p \rightarrow q$ $\neg p$
$T$ $T$ $T$ $F$
$T$ $F$ $F$ $F$
$F$ $T\checkmark$ $T\checkmark$ $T\checkmark$
$F$ $F\times$ $T\checkmark$ $T\checkmark$
Conclusion Premise 1 Premise 2

In the 4th case, the conclusion is false when both premises are true.
Argument is invalid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \rightarrow q) \land \neg p] \rightarrow q$
$p$ $q$ $p \rightarrow q$ $\neg p$ $(p \rightarrow q) \land \neg p$ $[(p \rightarrow q) \land \neg p] \rightarrow q$
$T$ $T$ $T$ $F$ $F$ $T$
$T$ $F$ $F$ $F$ $F$ $T$
$F$ $T$ $T$ $T$ $T$ $T$
$F$ $F$ $T$ $T$ $T$ $F$

The formula is not a tautology.
Argument is invalid by Formula

(15.) Premise: If a corrosive is acid, then it is dangerous.
Premise: Salt is not dangerous.
Conclusion: Salt is not a type of acid.


Let:
$p$: Salt is a type of acid.
$q$: Salt is dangerous.

$ Premise\: 1: p \rightarrow q \\[2ex] Premise\: 2: \neg q \\ \rule{2.5in}{0.5pt} \\ Conclusion\: \therefore \neg p \\[3ex] $ First Method: Valid and Invalid Forms of Arguments
Argument is valid by Modus Tollens

Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $p \rightarrow q$ $\neg q$ $\neg p$
$T$ $T$ $T$ $F$ $F$
$T$ $F$ $F$ $T$ $F$
$F$ $T$ $T$ $F$ $T$
$F$ $F$ $T\checkmark$ $T\checkmark$ $T\checkmark$
Premise 1 Premise 2 Conclusion
In the case where both premises are true, the conclusion is also true.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \rightarrow q) \land \neg q] \rightarrow \neg p$
$p$ $q$ $p \rightarrow q$ $\neg p$ $\neg q$ $(p \rightarrow q) \land \neg q$ $[(p \rightarrow q) \land \neg q] \rightarrow \neg p$
$T$ $T$ $T$ $F$ $F$ $F$ $T$
$T$ $F$ $F$ $T$ $F$ $F$ $T$
$F$ $T$ $T$ $F$ $T$ $F$ $T$
$F$ $F$ $T$ $T$ $T$ $T$ $T$
The formula is a tautology.
Argument is valid by Formula

(16.)


(17.) Mary sings soprano or alto or both.
Mary sings alto or tenor or both.
Therefore, Mary sings soprano or tenor.


Let:
$p$: Mary sings soprano.
$q$: Mary sings alto.
$r$: Mary sings tenor.

$ Premise\: 1: p \lor q \\[2ex] Premise\: 2: q \lor r \\[2ex] \rule{2.5in}{0.5pt} \\ Conclusion \therefore p \lor r \\[3ex] $ Second Method: Definition
Let us draw a truth table of the premise and the conclusion.
$p$ $q$ $r$ $p \lor q$ $q \lor r$ $p \lor r$
$T$ $T$ $T$ $T\checkmark$ $T\checkmark$ $T\checkmark$
$T$ $T$ $F$ $T\checkmark$ $T\checkmark$ $T\checkmark$
$T$ $F$ $T$ $T\checkmark$ $T\checkmark$ $T\checkmark$
$T$ $F$ $F$ $T$ $F$ $T$
$F$ $T$ $T$ $T\checkmark$ $T\checkmark$ $T\checkmark$
$F$ $T$ $F$ $T\checkmark$ $T\checkmark$ $F\times$
$F$ $F$ $T$ $F$ $T$ $T$
$F$ $F$ $F$ $F$ $F$ $F$
Premise 1 Premise 2 Conclusion
In the 6th case, the conclusion is false when both premises are true.
Argument is invalid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2)] \rightarrow conclusion$
$[(p \lor q) \land (q \lor r)] \rightarrow (p \lor r)$
$p$ $q$ $r$ $p \lor q$ $q \lor r$ $(p \lor q) \land (q \lor r)$ $p \lor r$ $[(p \lor q) \land (q \lor r)] \rightarrow (p \lor r)$
$T$ $T$ $T$ $T$ $T$ $T$ $T$ $T$
$T$ $T$ $F$ $T$ $T$ $T$ $T$ $T$
$T$ $F$ $T$ $T$ $T$ $T$ $T$ $T$
$T$ $F$ $F$ $T$ $F$ $F$ $T$ $T$
$F$ $T$ $T$ $T$ $T$ $T$ $T$ $T$
$F$ $T$ $F$ $T$ $T$ $T$ $F$ $F$
$F$ $F$ $T$ $F$ $T$ $F$ $T$ $T$
$F$ $F$ $F$ $F$ $F$ $F$ $F$ $T$
The formula is not a tautology.
Argument is not valid by Formula

(18.)


(19.) It is wrong to smoke in public if secondary cigarette smoke is a health risk. If secondary cigarette smoke were not a health risk, the American Lung Association would not say that it is. The American Lung Association says that secondary cigarette smoke is a health risk. Therefore, it is wrong to smoke in public.
Use p to represent "Secondary cigarette smoke is a health risk," use q to represent "It is wrong to smoke in public," and use r to represent "The American Lung Association says that secondary cigarette smoke is a health risk."


Let:
$p$: Secondary cigarette smoke is a health risk
$q$: It is wrong to smoke in public
$r$: The American Lung Association says that secondary cigarette smoke is a health risk

$ Premise\: 1: p \rightarrow q \\[2ex] Premise\: 2: \neg p \rightarrow \neg r \\[2ex] Premise\: 3: r \\[2ex] \rule{2.5in}{0.5pt} \\ Conclusion \therefore q \\[3ex] $ Second Method: Definition
Let us draw a truth table of the premises and the conclusion.
$p$ $q$ $r$ $p \rightarrow q$ $\neg p$ $\neg r$ $\neg p \rightarrow \neg r$
$T$ $T$✓ $T$✓ $T$✓ $F$ $F$ $T$✓
$T$ $T$ $F$ $T$ $F$ $T$ $T$
$T$ $F$ $T$ $F$ $F$ $F$ $T$
$T$ $F$ $F$ $F$ $F$ $T$ $T$
$F$ $T$ $T$ $T$ $T$ $F$ $F$
$F$ $T$ $F$ $T$ $T$ $T$ $T$
$F$ $F$ $T$ $T$ $T$ $F$ $F$
$F$ $F$ $F$ $T$ $T$ $T$ $T$
Conclusion Premise 3 Premise 1 Premise 2

In the case where all premises are true (only one case), the conclusion is also true.
Argument is valid by Definition

Third Method: Formula
Let us draw the truth table for the formula: $[(premise\: 1) \land (premise\: 2) \land (premise\: 3)] \rightarrow conclusion$
$[(p \rightarrow q) \land (\neg p \rightarrow \neg r) \land r] \rightarrow q$
$p$ $q$ $r$ $p \rightarrow q$ $\neg p$ $\neg r$ $\neg p \rightarrow \neg r$ $(p \rightarrow q) \land (\neg p \rightarrow \neg r)$ $(p \rightarrow q) \land (\neg p \rightarrow \neg r) \land r$ $[(p \rightarrow q) \land (\neg p \rightarrow \neg r) \land r] \rightarrow q$
$T$ $T$ $T$ $T$ $F$ $F$ $T$ $T$ $T$ $T$
$T$ $T$ $F$ $T$ $F$ $T$ $T$ $T$ $F$ $T$
$T$ $F$ $T$ $F$ $F$ $F$ $T$ $F$ $F$ $T$
$T$ $F$ $F$ $F$ $F$ $T$ $T$ $F$ $F$ $T$
$F$ $T$ $T$ $T$ $T$ $F$ $F$ $F$ $F$ $T$
$F$ $T$ $F$ $T$ $T$ $T$ $T$ $T$ $F$ $T$
$F$ $F$ $T$ $T$ $T$ $F$ $F$ $F$ $F$ $T$
$F$ $F$ $F$ $T$ $T$ $T$ $T$ $T$ $F$ $T$
Tautology

The formula is a tautology.
Argument is valid by Formula

(20.)






Top




(21.)


(22.)


(23.)


(24.)


(25.) No doctor is poor.
Some teachers are poor.
Therefore, some teachers are not doctors.


Let:
set of doctors = D
set of poor people = P
set of teachers = T

Number 25

Premise 1: No doctor is poor means D and P are disjoint sets
Premise 2: Some teachers are poor means T and P have some elements in common...intersection of sets
Conclusion: Therefore, some teachers are not doctors.

Because some teachers are poor, this means that those teachers that are poor, are not doctors. This is because no doctor is poor. This is represented by the shaded area in the diagram (one of the possible Euler diagrams).
Other possible Euler diagrams must include that shaded area.
Hence, the conclusion follows from the premises.
The argument is valid.

Would you like further explanations?
Let us draw all the possibilities (cases) for Premise 1 and Premise 2

Let:
set of doctors = D
set of poor people = P
set of teachers = T

Number 25-1

These are the two cases for Premise 1 and Premise 2
In the 1st case, some teachers are doctors and some teachers are not doctors.
In the 2nd case, no teacher is a doctor.
However, this does not contradict the conclusion that some teachers are not doctors.
The argument is valid.

(26.)


(27.)


(28.)


(29.) All policemen are rude.
Nobody who is meticulous is a policeman.
Therefore, rude people are not meticulous.


Let:
set of policemen = P
set of meticulous people = M
set of rude people = R

Number 29

Premise 1: All policemen are rude means P is a subset of R
Premise 2: Nobody who is meticulous is a policeman means M and P are disjoint sets
Conclusion: Therefore, rude people are not meticulous.

Based on the diagram (one of the possible Euler diagrams), it is possible that some rude people are meticulous because one of the options is the shaded area: which represents the set that there is at least one rude person that is meticulous.
Hence, the conclusion does not follow from the premises.
The argument is invalid.

Would you like further explanations?
Let us draw all the possibilities (cases) for Premise 1 and Premise 2

Number 29-1

These are the three cases for Premise 1 and Premise 2
In the 1st case, rude people are meticulous.
In the 2nd case, some rude people are meticulous.
In the 3rd case, no rude person is meticulous.
Because of the 1st Case and the 2nd Case, the argument is invalid.

(30.)


(31.) All politicians are rich.
Rich people are never happy.
Therefore, no politician is happy.


Let:
set of politicians = P
set of rich people = R
set of happy people = H

Number 31

Premise 1: All politicians are rich means P is a subset of R
Premise 2: Rich people are never happy means R and H are disjoint sets
Conclusion: Therefore, no politician is happy.

Based on the diagram, it is evident that no politician is happy because P and H are also disjoint.
Hence, the conclusion follows from the premises.
The argument is valid.

(32.)


(33.)


(34.)


(35.)


(36.)